Variations of Gravity

The Variations of Gravity are the supposed faint variations to gravity caused by the earth, moon and sun. Some precision devices are alleged to detect these variations, while other precision devices are unable to do so.

The Gravimeter

On analysis of the gravimeter we find that the device is not directly measuring gravitational acceleration at all. It is measuring noise and using many software algorithms to interpret and filter out the noise. Every part of the device's components—the lasers which measure the motion, the mirrors, the springs which launch the mass up and down the small tube, and all component parts—are all connected in some manner to the earth, and are subject to noise and vibrations.

https://link.springer.com/chapter/10.1007/978-3-662-03482-8_9

  “ One of the most serious problems for absolute gravimeters is the vibration disturbances. ”

Marine Gravimeters

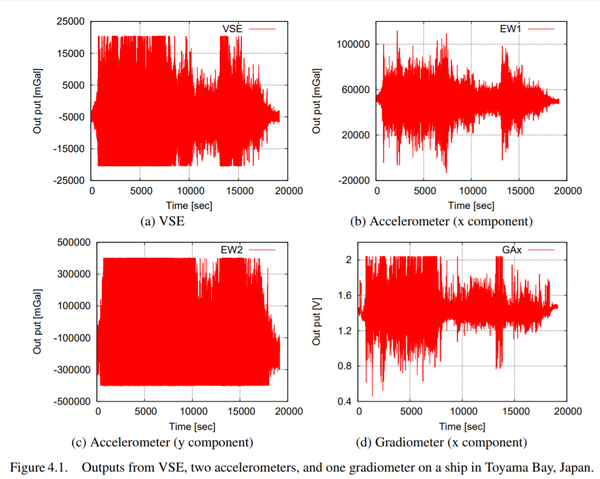
Below we see an example of gravimetry devices and methods for marine gravimetric surveying:

http://www.iitk.ac.in/nicee/wcee/article/WCEE2012_1237.pdf

An example is given of outputs from VSE, Accelerometer, and Gradiometer devices on a mid-size ship navigating Toyama Bay, Japan:

Following the illustration we find the following:

  “ FINDING GRAVITY FROM VERY NOISY DATA

In the previous section, the low-pass filter is used to find the gravity from the noisy data. We can expect that the variation of gravity consists of components with long period, however the vibration of carrier with short period. If this expectation is satisfied, the low-pass filter should work well. Actually, analysis for the first observation on an observation wheel works well, though, not for the other two observations. The reason is the difference of the amplitude of the noise: it is very difficult to pick up the gravity data with very small amplitude from very large vibration of the carrier. This must be limitation of simple filtering technique.

To reduce the vibration of the carrier, we can choose two different approaches. ”

From the above we see that a need to use algorithms and filters to 'find gravity' from noise. The levels of g are not readily apparent, and must be constructed by subtracting from the noise to 'find gravity'. The above passage states that "We can expect to find the variations of gravity consists of components with long period, however the vibration of the carrier with short periods."

The reader may decide whether the process of subtracting vibrations with one characteristic to reveal other vibrations is truly measuring gravity. Why does "gravity" need to be found?

Galathea-3: A global marine gravity profile

The Danish vessel Galathea-3 describes its methods for gravimetric surveying:

Galathea-3: A Global Marine Gravity Profile

  “ The Galathea-3 navigation data included raw depth records provided by The Danish Hydrological Office. The data transfer was sometimes unstable and the data themselves were often very noisy. A preparation of such data for the Bouguer gravity processing requires a cumbersome data cleaning the removal of the spikes, the smoothing, and often the interpolation ”

We see that the process involves extensive data analysis, filtering, and cleanup from noise — to 'find gravity'.

Airborne Gravimetry

Airborne gravimetery methods reviel the same:

Improving the Accuracy and Resolution of SINS/DGPS Airborne Gravimetry

  “ Because the resulting signal is very noisy (see Sections 2.3.2 to 2.3.4 for details), its derivation is followed by a filtering step that removes most of the noise by low-pass filtering the data. ”

Again, we see that filtering is necessary to clean up the noise.

General/Land Based Gravimetry

The following paper describes the process of turning a laser signal into a "variation of gravity":

Ultra-high Precision, Absolute, Earth Gravity Measurements

The paper describes that the fringe signal from the laser beam is digitized and processed:

  “ One of the new features of our setup is that the whole fringe signal (with up to 1.6 million data points) is digitized and processed. In other gravimeters usually just parts of the fringe signal are used for data processing. Since the duration of our FB’s fall in the MPG-1 is about 200 ms, the resultant signal reaches a frequency of up to 6.2 MHz. As an industry standard, a sampling rate of at least 7 times the measured frequency is suggested. ”

Processing Steps

The fringe signal from the laser must be filtered with software:

The remainder of the document describes how the filtering occurs. All sources, to the best of the author's ability, are given an "uncertainty budget," to which is subtracted from the noise. The uncertainty budgets are estimated ranges to which a phenomenon may be contributing to the noise. All possible phenomena in nature must be considered and precisely defined. Everything from air drag, electrostatic fields, pressure, seismic vibrations, &c.

In the Table of Contents we find a list of tables, showing the various elements which are subtracted:

4.6 Uncertainty budget of the measured imbalance – Method II. . . . 84
4.7 Uncertainty budget: COM and OC adjusted – Method II. . . . . . 84
5.1 Uncertainty budget for air drag . . . . . . . . . . . . . . . . . . . 89
5.2 Uncertainty budget for outgassing . . . . . . . . . . . . . . . . . . 90
5.3 Uncertainty budget for eddy currents . . . . . . . . . . . . . . . . 91
5.4 Uncertainty budget for electrostatic field . . . . . . . . . . . . . . 91
5.5 Uncertainty budget for instrumental masses . . . . . . . . . . . . 94
5.6 Uncertainty budget for laser verticality . . . . . . . . . . . . . . . 96
5.7 Length standard specifications. . . . . . . . . . . . . . . . . . . . 96
5.8 Uncertainty budget for laser stability . . . . . . . . . . . . . . . . 97
5.9 Frequency standard specifications. . . . . . . . . . . . . . . . . . . 98
5.10 Uncertainty budget for clock stability . . . . . . . . . . . . . . . . 98
5.11 Uncertainty budget for corner cube rotation . . . . . . . . . . . . 98
5.12 Uncertainty budget for radiation pressure . . . . . . . . . . . . . . 99
5.13 Uncertainty budget for beam divergence . . . . . . . . . . . . . . 100
5.14 Uncertainty budget for temperature gradient . . . . . . . . . . . . 101
5.15 Uncertainty budget for seismic noise . . . . . . . . . . . . . . . . 107
5.16 Uncertainty budget for speed of light . . . . . . . . . . . . . . . . 108
5.17 Uncertainty budget for effective height . . . . . . . . . . . . . . . 109
5.18 Specifications of Photoreceiver. . . . . . . . . . . . . . . . . . . . 110
5.19 Uncertainty budget for amplifier . . . . . . . . . . . . . . . . . . . 111
5.20 Uncertainty budget for solid Earth tides . . . . . . . . . . . . . . 113
5.21 Uncertainty budget for ocean loading . . . . . . . . . . . . . . . . 113
5.22 Uncertainty budget for polar motion . . . . . . . . . . . . . . . . 114
5.23 Uncertainty budget for environmental pressure . . . . . . . . . . . 115
5.24 Uncertainty budget for Coriolis force . . . . . . . . . . . . . . . . 116
5.25 Uncertainty budget for MPG-1. . . . . . . . . . . . . . . . . . . . 117
5.26 Uncertainty budget for MPG-2. . . . . . . . . . . . . . . . . . . . 118

Once all of these items and their theoretical uncertainties are subtracted, we are left with "gravity".

Gravimeter Tides

The above pdf mentions that long-term analysis of the gravimeter noise can detect the tides:

The main conclusion from this is that the tides may be related in some manner to vibration or noise that is being processed. No mechanism is presented, or is identifiable, from a long term analysis of vertical vibration trends.

Fringe Noise

We look at the following paper:

Comparison of three digital fringe signal processing methods in a ballistic free-fall absolute gravimeter

From the introduction the author states that the input is a noisy fringe signal.

  “ The advantages of digitizing the entire fringe signal are as follows: the imperfect electronic components are eliminated; the full information on the quality of the fringe signal is available; a noisy fringe signal is digitally filtered at the pre-processing steps; different numerical algorithms to calculate a g value can be interchangeably applied. ”

The g value must be extracted with various methods. It is not direct and apparent:

  “ Usually, the fringe signal is digitized at a sampling rate exceeding its maximum frequency. However, a few methods have demonstrated the possibility to extract a g value from the fringe signal sampled far below the Nyquist criterion (table 1). ”

Multiple methods, functions, to "extract" the gravity value from the "noisy fringe signal."

Not an obvious and apparent method; multiple models are involved:

  “ This method has been developed by Niebauer et al [18]. The basic idea is to extract the gravity value directly from the undersampled waveform of the fringe signal given by the non-linear model (1). To find the initial estimates of the model parameters, required in the non-linear leastsquares adjustment, it was proposed to iteratively demodulate the digitized waveform. For this the acquired fringe signal is multiplied by a swept sinusoid, denoted as a complex heterodyne function. This method requires prior knowledge of the sweep parameters. A complex heterodyning is used to reduce the bandwidth of the demodulated signal in order to double the signal-to-noise ratio, compared with a singlesideband demodulation [18]. ”

Filtering agreement can be made with other fringe signal processing methods:

  “ Up to now, a few comparisons of different fringe signal processing methods have reported an agreement within several parts in 10^9 of g [10, 18] or even larger [12, 16]. ”

These processes are filtering out the noise to find trends. From the conclusion we read:

  “ 5. Conclusions

For the first time, a comparison of the three different digital fringe signal processing methods, realized in the same absolute gravimeter, has been completed. The two-sample zerocrossing method, the windowed second-difference method and the method of non-linear least-squares adjustment using the initial parameter estimates, found by the demodulation of the undersampled fringe signal, have been compared in numerical simulations, hardware tests and actual measurements with the MPG-2 absolute gravimeter. Up to now, diversity of the digital fringe signal processing methods in absolute gravimetry was mainly caused by a certain technical restriction: processing of the huge amount of digitized fringe data was limited by the available computing capabilities. Owing to this, several original, though complicated, algorithms have been developed. In contrast, the reported comparison involves the simple and straightforward method to detect all the zero-crossings in a digitized fringe signal. ”

This document was written in 2010. If Gravimetry were really 'direct measurement of the acceleration,' as some have claimed, then the reader might ask, why was it was limited by pre-2010 computing capabilities? The answer is that it is not direct at all. It is the many algorithms necessary to interpret the "noisy" fringe signal that need large amounts of computing power. The process is interpretation of a noisy fringe signal.

From the paper:

  “ Therefore, a combination of the different methods of fringe signal processing in the same instrument is a useful tool to compose a more complete uncertainty budget. ”

Uncertainty budgets, just as we saw in the earlier paper, are subtracted from the noise in the effort to find gravity.

Location Trends

Q. How is it that the level of g is different at high altitudes and at the poles?

A. The gravimeter measures fringe noise, not the "level of g". The output is the result of specialized filtering. That, after the final filtering and analysis, there may be trends at the top of a mountain, or in an environment which sits on a large amount of compressed ice, or with different environmental affects, should not be surprising. Gravity itself is not, and cannot be, identified from the above processes.

Here is an example of seismologists telling us that the North Pole is a very noisy environment, and acquiring useful seismology data is an issue:

https://polarforskningsportalen.se/en/arctic/expeditions/lomrog/cruise-reports/lomrog-det-danska-kontinentalsockelprojektet

  “ The crucial issue of seismic data acquisition in ice covered waters is to protect the seismic equipment from being damaged by sea ice while simultaneously acquiring useful data in this very noisy environment. ”

Like with gravimeters, numerous levels of filtering and processing is required.

The Torsion Balance

While the gravimeter relies on noise and vibration analysis, the types of experiments used in the equivalence principle tests are of a different sort. The equivalence principle tests are incredibly reliable precision machines which are used to measure the equivalence principal to increasing sensitivity.

Experimenters have redesigned the EP Torsion Balance tests to try and detect the gravity variations caused by the sun, moon, and the tidal forces. It was found that the gravitational influence of the sun, moon, or the tidal forces could not be measured as manifest of the attraction of the bodies in the experiments. Variations to "gravity" did not appear.

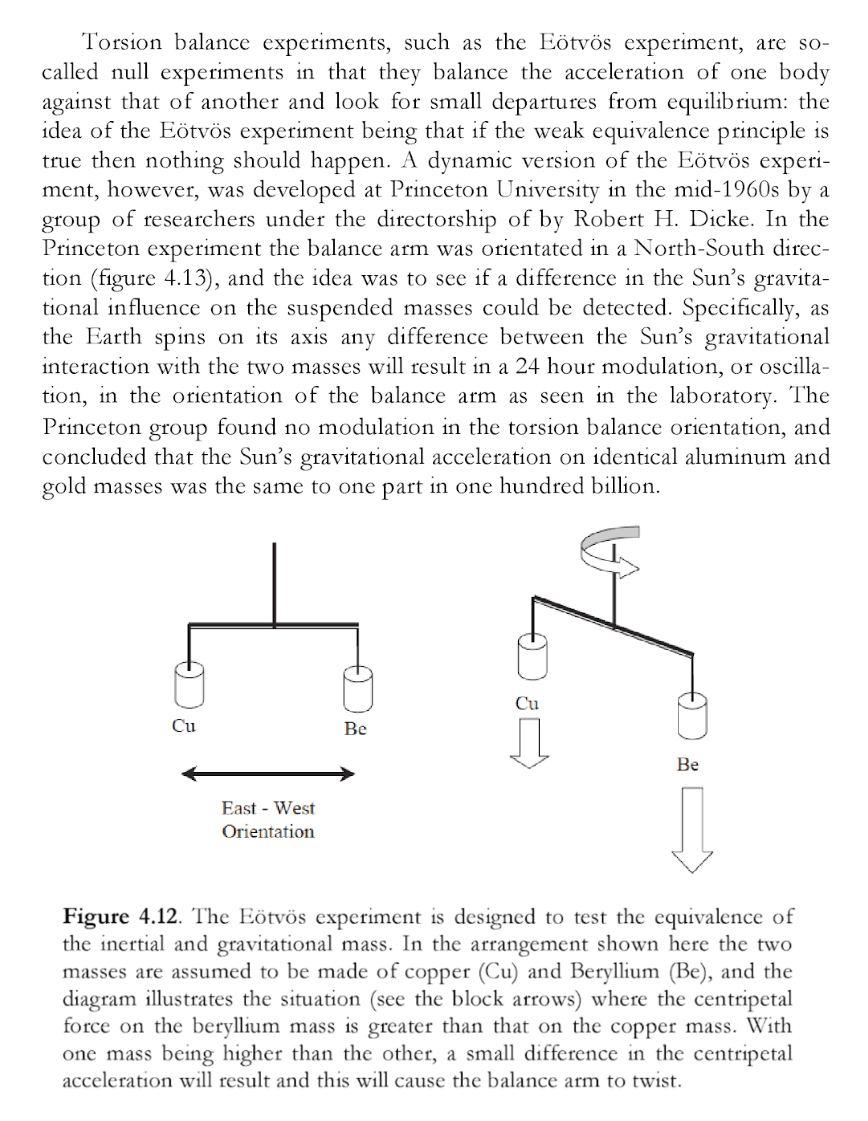
The Princeton Experiment

From 'The Pendulum Paradigm: Variations on a Theme and the Measure of Heaven and Earth', by Martin Beech, we read the following:

Essentially, the experiment is summarized as follows:

  “ In the Princeton experiment the balance arm was oriented in a North-South direction (figure 4.13), and the idea was to see if a difference in the Sun's gravitational influence on the suspended masses could be detected. Specifically, as the Earth spins on its axis and difference between the Sun's gravitational interaction with the two masses will result in a 24 hour modulation or oscillation, in the orientation of the balance arm as seen in the laboratory. The Princeton group found no modulation of the torsion balance, and concluded that the Sun's gravitational acceleration on identical aluminum and gold masses was the same to one part in one hundred billion. ”

The masses were not attracted to the sun in the experiment, to an accuracy of one part in one hundred billion.

Repetitions

Additional experiments of this class are described. The first experiment in this list is the Princeton experiment above:

Authors	Year	Description	Accuracy
Roll, Krotkov and Dicke	1964	Torsion balance experiment, dropping aluminum and gold test masses	difference is less than one part in one hundred billion
Branginsky and Panov	1971	Torsion balance, aluminum and platinum test masses, measuring acceleration towards the sun	difference is less than 1 part in a trillion (most accurate to date)
Eöt-Wash	1987–	Torsion balance, measuring acceleration of different masses towards the earth, sun and galactic center, using several different kinds of masses	difference is less than a few parts in a trillion

The Eöt-Wash experiments, which measured the acceleration of different masses towards the earth, sun, and galactic center was repeated by others:

https://plato.stanford.edu/entries/physics-experiment/app4.html

  “ The torsion-balance experiments of Eöt-Wash were repeated by others including (Cowsik et al. 1988; Fitch, Isaila and Palmer 1988; Adelberger 1989; Bennett 1989; Newman, Graham and Nelson 1989; Stubbs et al. 1989; Cowsik et al. 1990; Nelson, Graham and Newman 1990). These repetitions, in different locations and using different substances, gave consistently negative results. ”

See Also

• Universal Acceleration
• Evidence for Universal Acceleration
• The Cavendish Experiment