Moon Tilt Illusion

If the Moon is illuminated by the Sun it is expected that its illuminated portion should always point at the Sun. The Moon Tilt Illusion is a phenomenon in which the lit portion of the Moon unexpectedly points away from the Sun. The phenomenon is most extreme for phases between Half Moon and Full Moon. The Moon Tilt Illusion even occurs after the Sun has set, a situation in which the the illuminated portion of the Moon is pointing upwards and away from the Earth while the Sun is below the horizon.

Some attribute this phenomenon to 'perspective', while astronomers tell us that there is a 'celestial sphere' which the celestial bodies glide upon at different angles, and upon which straight lines become curved. We are given a series of analogies and explanations separate from, and incompatible with, the Round Earth Theory.

The Moon Tilt Illusion is geometric anomaly predicted by the Flat Earth Theory. Under the FET the Moon Tilt is directly predicted by Electromagnetic Acceleration (EA). The nature of EA results in the tilt of the Moon's phase away from the Sun and, for those who will accept the proof when furnished, acts as a vivid piece of evidence for the Flat Earth celestial model which is visible and accessible to all.

Description

(Source)

Professor Myers at the University of Pennsylvania provides the following description:

http://www.upenn.edu/emeritus/essays/MyersMoon.html

Moon Tilt Illusion sketch provided by Professor Myers

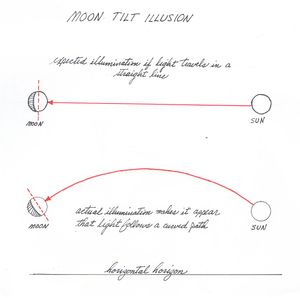
  “ One evening several years ago, I took this picture of the misty glow of an almost full moon shining between pine trees in my backyard. The moon was beautifully illuminated and dominated the dark sky. Yet something was disturbing about the atmospheric night scene: the illumination of the moon seemed to be coming from the wrong direction! In this photograph of a waxing moon in the southern sky, its illumination appears to be coming from above to the right. But the sun---which had set an hour earlier---was already below the western horizon to the right when this photo was taken. If the sun is below the horizon, I thought, shouldn't its illumination of the moon appear to be coming from below the horizon? Intrigued, I made further observations when both the sun and the moon occupied the evening sky at the same time, and it certainly seemed that light rays from the sun would have to follow a curved path to shine on the moon at the observed angle. The sketch drawn below may help to explain the difference between what I expected to see and what I actually saw.

I asked everyone willing to listen if they were familiar with this illusion: why does a light ray from the sun to the moon appear to follow a curved path? No one had seen or heard of it. Several imaginative explanations were offered: "The light rays are bent by the earth's atmosphere." Or, "Gravitational lensing as predicted by Einstein's general theory of relativity is responsible." I was surprised not to find the illusion described in astronomy textbooks. I googled "moon illusion" but the articles were about an entirely different illusion: the apparent magnification of the full moon when it's close to the horizon.

Finally, help came from my daughter, who located published papers by googling "moon tilt illusion". The scientific explanation is based on the projection of a straight line onto the surface of a sphere. A simpler explanation was provided in a conversation with Benjamin Shen, Professor Emeritus of Astronomy at Penn, who said that light appears to follow a great circle route from the sun to the moon. That's why the moon's lit face appears to us not to face the sun squarely, regardless of whether the sun is above or below the horizon.

The moon tilt illusion is counter-intuitive and magical---look for it the next time you are under a night sky. ”

Moon Tilt Observational Diagram

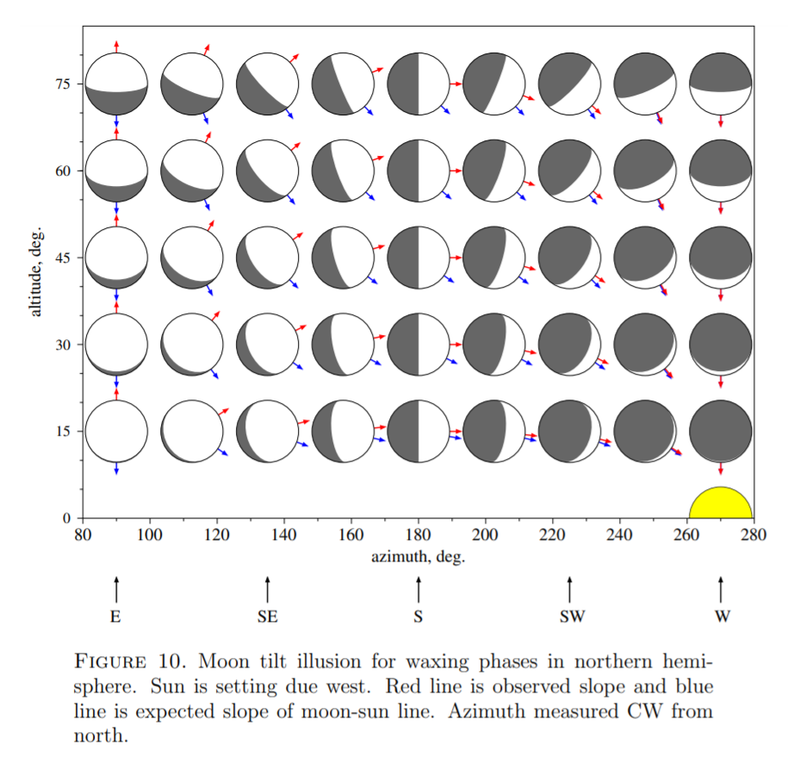
In a related paper from the same author titled The Moon Tilt Illusion (Archive), Professor Myers provides the following diagram of the observed tilt of the Moon and its displacement from the expected angle for waxing phases in the North:

Altitude is the elevation of the moon in the sky. Azimuth is the degrees clockwise from North. The diagram shows phases from full to new. At times the Moon is pointing towards the Sun, and at other times the Moon is pointing away from it. When opposite from the Sun, the Moon's tilt can be wildly displaced from its expected position.

Other Versions

• Moon Tilt Northern Waning
• Moon Tilt Southern Waxing
• Moon Tilt Southern Waning

From the source:

  “ The most impressive illusion occurs at sunset when the gibbous moon is at high altitude in the southwest or at sunrise when the gibbous moon is at high altitude in the southeast (both cases for the northern hemisphere). ”

Lunar Phases at Sunset

Another depiction of the various orientations of the Moon in relation to the Sun at the time of sunset for waxing phases in the North may be found below:

(Source)

Other Versions

• Waning Phases at Sunrise

Sun and Moon Anomaly

From a Stack Exchange post on the Moon Tilt Illusion we find an observation (Archive):

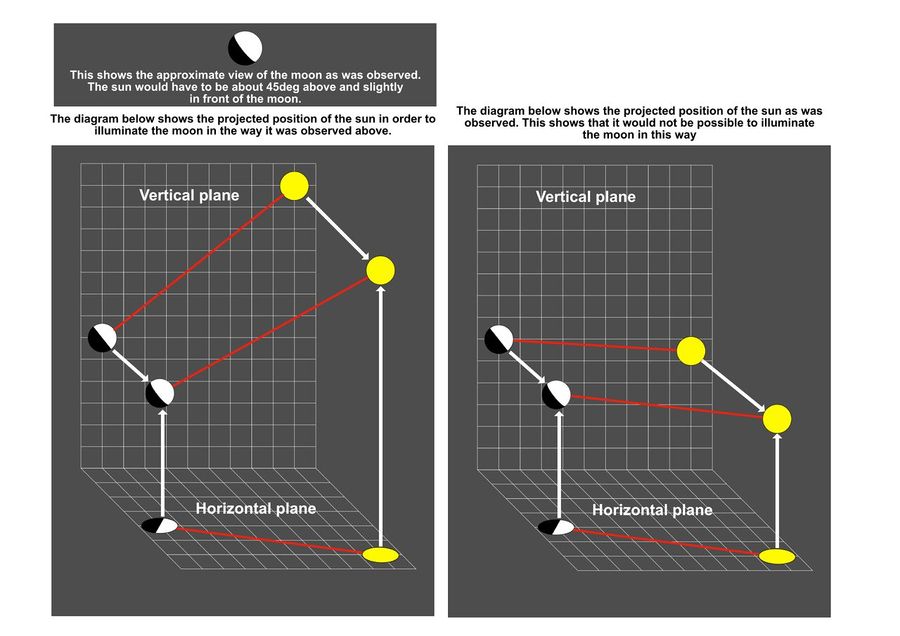
  “ Below is a photo that my son took in Scotland showing the sun and moon at the same time. I immediately noticed this anomaly that the light illuminating the moon could not possibly come from the sun. I sent the photo to 4 University astronomy departments and only one responded and that was Cambridge University which is near where I live. The response came from the department librarian (not an astronomer) who said he had never heard of this before. He gave me two possible solutions, one was was from an engineer (not an astronomer) in which he got confused between perspective and light ray tracing and the other was referring to Einstein's theory of light bending by gravity. I check out Einstein and the effect was so small as to be almost immeasurable. ”

  “ I have looked at the various 'complex' explanations for what to me is a very simple model. What need is there to introduce 'curved planes' and 'starry sky domes' all of which do not exist in reality? It is only referred to as an 'illusion' because observation doesn't fit the conventional model hence the complex explanations to try and make it work. The anomaly is acknowledged to exist with or without photos. Since everyone believes that the moon is illuminated by the sun then simple normal physics do not seem to work. Either the physics is wrong or the sun does not illuminate the moon. I realise that is a heavy statement!

Therefore I state once again:

1. The sun and the moon are two objects (like a torch and a football) that are suspended in a 3 dimensional space and size should not matter.
2. The moon/football are illuminated by the sun/torch and a perpendicular line or light ray can be drawn between them.
3. It doesn't matter where in space you choose to view them, a perpendicular line or light ray can still be drawn between them.

This drawing explains my doubts: ”

  “ I'm very surprised that some of you have never noticed it before hence the suggestion asking me to post a video. This is a very common occurrence and I have seen it many many times as I go for my morning walk at about 8.00am every morning. I have never thought of actually tabulating my observations. ”

Flat Earth Moon Tilt

It has been asked if there is an example of a celestial event that the Flat Earth Theory predicts which the Round Earth Theory does not. The Moon Tilt Illusion is one such example. While RET has difficulty explaining this anomaly, with its professors calling it "counter-intuitive and magical"^[1], and multiple contradictory and geometrically questionable explanations are put forward in attempt to explain it, the phenomenon is directly predicted by the Flat Earth astronomical model. According to the theory of Electromagnetic Acceleration light curves upwards over very long distances, is the cause for the rising and setting of celestial bodies, and is responsible for various dome-like observations. When this scheme of upwardly bending light is applied to the orientation of the Moon it is seen that Electromagnetic Acceleration directly predicts the Moon Tilt Illusion.

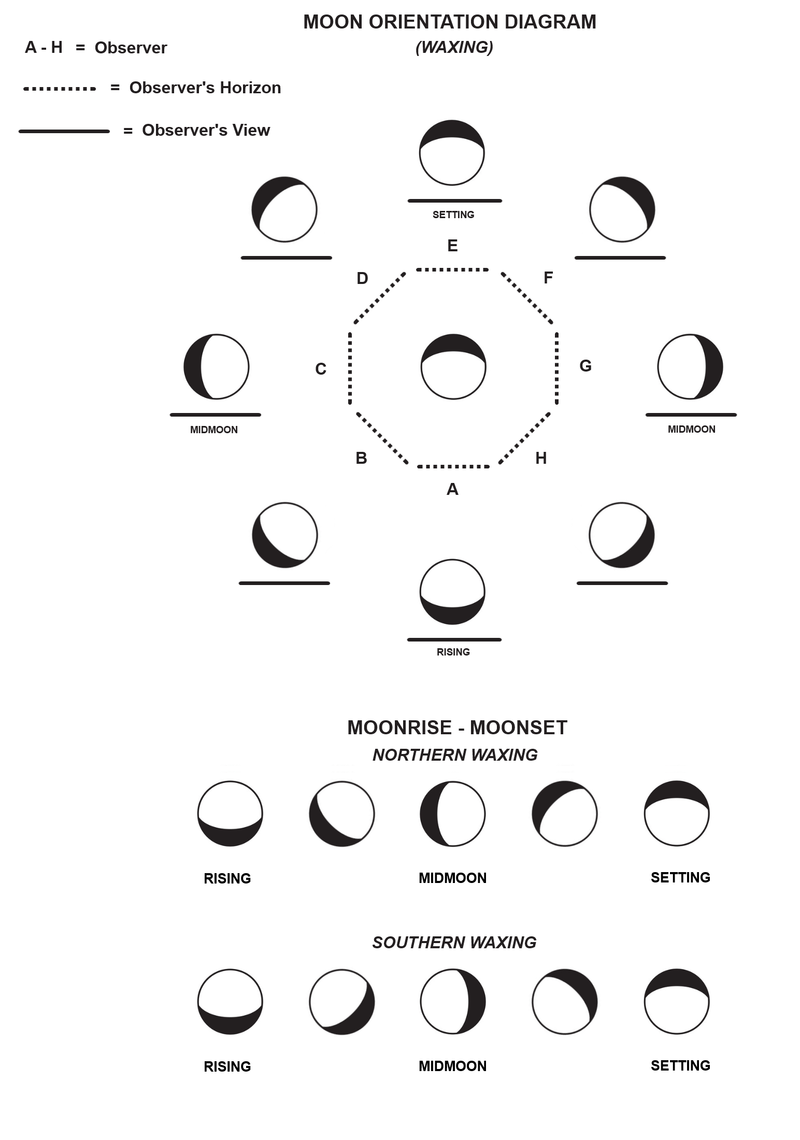
Moon Flipped

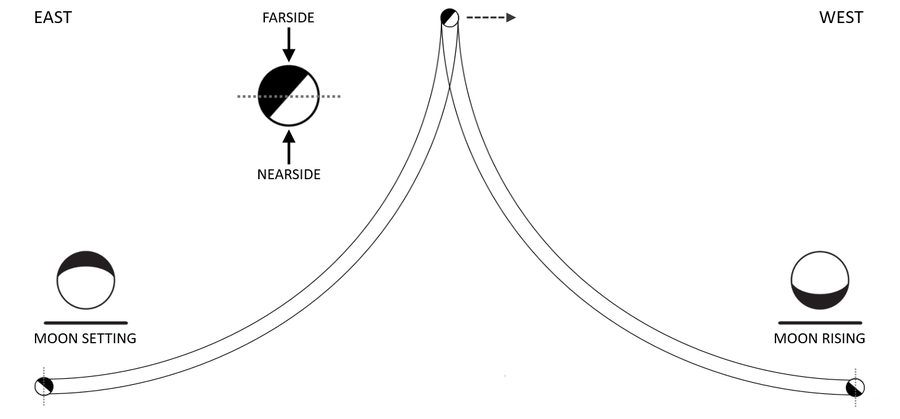
Due to EA the observer will always see the nearside (underside) of celestial bodies. As result the orientation of the image on opposite sides will be upside-down. The illustration below shows the extremes of the Moon's rising and setting and the upside-down flipping of the Moon's image to observers positioned on opposite sides of the Moon.

An observer to the West of the Moon sees the phase pointed upwards away from the horizon, and an observer to the East of the Moon sees the phase pointed downwards towards the horizon.

Moon Tilted

When viewing the Moon at various positions around it, it is seen that the Moon's phase tilts until it matches the opposite orientation. In the below illustration observers A - H are standing around the Moon, viewing it at various positions around it. The Moon over the solid horizon shows the observer's view.

Notice that the views on opposite sides of each observer (A - H) are vertically flipped to each other like in the rising and setting side view diagram.

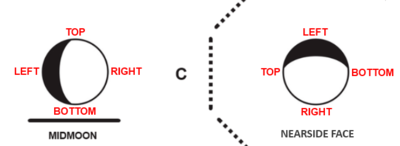
To simulate the view for each observer flip the central nearside face in relation to the observer's orientation around the Moon (positions A - H), so that the observer sees the furthest part of the Moon from his or her position at the closest to that observer's horizon. This represents each observer's view of the Moon.

For instance, Observer C at midmoon is looking to the right (Southward) at the Moon and from that position will see the darkened portion to the left of the Moon's image, with the furthest part of the Moon from Observer C's location at the bottom closest to that observer's horizon. The same may be applied for each observer, relative to their various positions around the Moon. Alternatively, one may take the vertically flipped rising phase for Observer A and rotate that image in 45 degree increments to match the positions of the Observers B - H around the Moon.

EA predicts that between rising and midmoon the Moon's phase will be pointed significantly away from the Earth and Sun, angled upwards above it. At midmoon the illuminated portion of the Moon will be pointing at a right angle in the sky. Between midmoon and setting the phase will be pointing downwards towards the Earth. EA also predicts that the Moon's face will roll and rotate clockwise in the North and counter-clockwise in the South.

Comparison

Compare the EA Moon Tilt diagrams to the diagrams shown at the beginning of the page showing the Moon Tilt Illusion for the Waxing Phases in the North and South.

• Moon Tilt Northern Waxing
• Moon Tilt Southern Waxing
• Northern Waxing Phases at Sunset

Round Earth Explanations

Perspective Explanations

An explanation of the Moon Tilt Illusion for the Round Earth Theory is given in the form of a perspective effect. It is possible to arrange yourself under an object do that it points upwards above your head. It is claimed that this is occurring with the Moon.

Scene zoomed out:

Simultaneous Objects

One issue with this explanation of perspective is that if the observer is ever in a position to see both the Moon and Sun simultaneously, the illuminated portion Moon should point at the Sun. When moving the camera around the above scene, whenever the green arrow and yellow ball are in the same frame, the arrow points at the ball along that straight line.

Yet, we find that in the Moon Tilt Illusion is possible for an observer to see both the Moon and Sun simultaneously, misaligned to each other.

From http://www.astropix.com/html/l_story/moonill.html (Archive) an astrophotographer reports:

  “ Now, I have always under the impression that if you took the Moon's phase illumination angle it would draw a line straight back to the sun. But this sure wasn't what I thought I saw this day.

Obviously, it's an illusion that has something to do with a three-dimensional space being projected onto a two-dimensional plane in my eyeballs. Some people have tried to explain it as involving great circles, just as airplanes fly great circle routes to places on the opposite side of the globe. However they only do this because they can't fly a straight line through the Earth.

What I can't seem to get past is that the Sun and the Moon were in the same field together and I could view them both at the same time and that the light from the Sun is going in a straight line from the Sun to the Moon. It is not following a great circle. ”

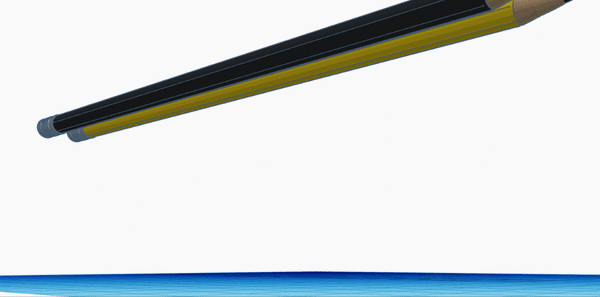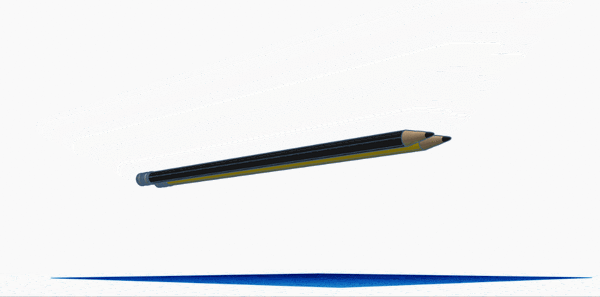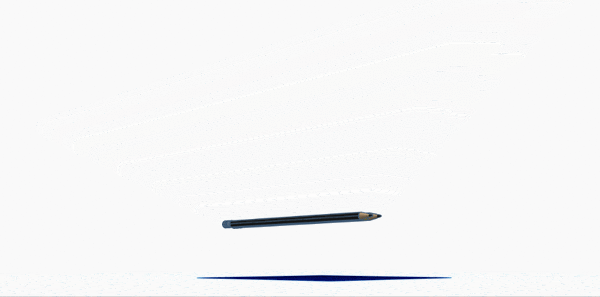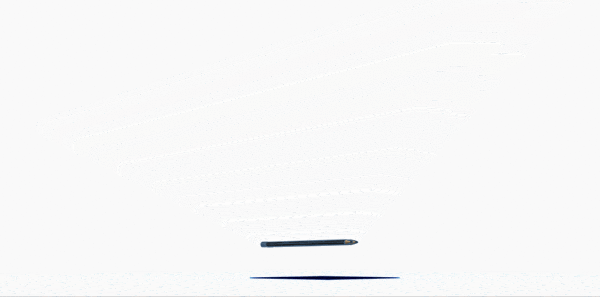
Pencil Example

Another potential issue is that perspective affects bodies less with greater distance. In the below example a pencil is affected less and less with greater distance. In the below simulations the pencils are horizontal and parallel to the floor surface. At close proximity the pencils seem to be angled upwards due to a perspective effect, despite the parallel nature of the pencils to the floor. Perspective causes the pencils to point upwards. Receding backwards away from the scene causes the pencils to even out as they become distant.

(Tinkercad Pencil Model Source)

Some have argued that the Moon Tilt Illusion is caused by such a perspective effect, which causes the illuminated portion of the Moon to tilt and point upwards. Yet, we see from the above that this is only a close range effect.

Consider the following: If the pencils recede into the distance until they are at the distance of the RE Moon, presuming that we could see them at that distance, will the pencils point upwards?

Vertical Displacement

It is agreed by all parties that if the pencils recede into the distance until they are at the distance of the RE Moon, that they will not point upwards. The implication being that if the Moon were distant from the observer, it would not naturally tilt to perspective. In response, our opponents reply "The illusion is caused by a combination of the lateral and vertical displacements of the observer to the moon." We are asked to lift the distant pencils in altitude and to observe how they behave. We do so below:

The pencils give important cues to what is occuring to objects when modified by perspective. When the pencils at the bottom positions are level with the observer they do not tilt. When the pencils are risen in altitude they do tilt. At the top position we see the underside of the pencils, as the pencils point upwards. This is suggested to be what is happening with the Moon. If one compares the Moon Tilt Observational Diagrams to the upwardly pointing pencil, there are similarities with the upwardly pointing phase.

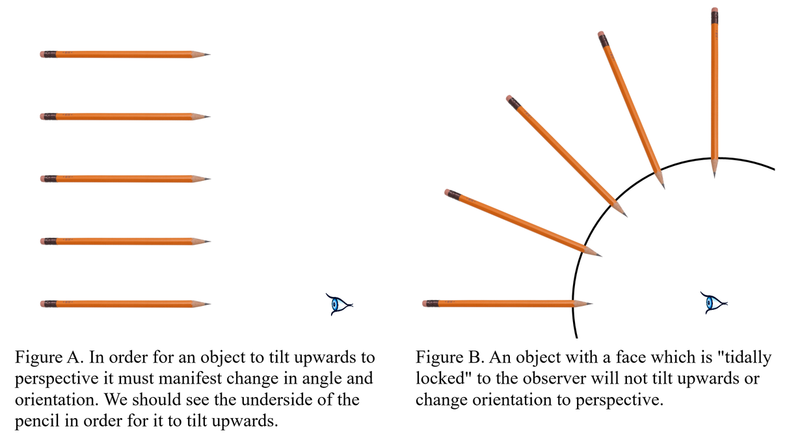
In order for the pencils to tilt upwards in this manner they must be changing orientation. When the pencils are at the top position and pointing upwards we are looking at the underside of the pencils. However, when Moon rises in altitude from the horizon do we ever see different sides of the Moon?

We regret that the answer in the negative. We observe the same nearside face of the Moon at all times, regardless of how it is lit. The Moon never tilts in orientation. According to RE this is because the Moon is tidally locked to the Earth and the Moon's nearside face is pointing at the Earth at all times. FE explains why we always see the nearside of the Moon as a result of Electromagnetic Acceleration. In order for the Moon to tilt upwards in this perspective explanation the Moon would need to manifest a change in orientation. We should see it rotate upwards in order to see it tilt upwards. We should see the Moon from another angle, just like the pencils in the above animation, or like when lifting any body in front of us. As we always see the same side of the Moon, this is a contradiction. The Moon does not change angle to perspective. Its nearside face points at the observer at all times.

Difference in Position Diagram

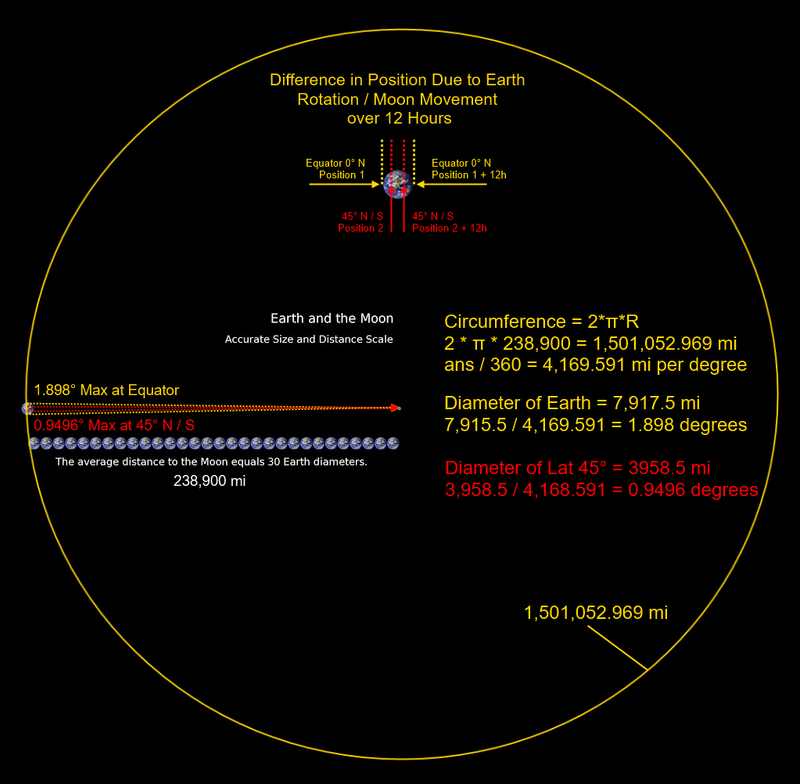
According to the RET over the course of a day the changing perspective of the Moon to an observer on Earth is negligible. The Moon maintains its distance from the center of the Earth at all times, with its nearside face tidally locked to the Earth as the Moon slowly rotates around it. The Earth, in turn, rotates diurnally at a faster rate in relation to the Moon. An observer on the surface of the Earth at either side of the Equator will observe a change in the position of the Moon by less than 2 degrees due to positional changes alone, as demonstrated in the below top-down illustration:

In the above diagram the outer orange circle is the distance between the Earth to the Moon turned into a circle. The total distance around that circle in miles by 360 degrees gives the number of mile per degree, which is used to find the displacement in degrees between the extremes of either side of the Earth. We see that the displacement in degrees is minimal.

Diurnal Liberation

Wikipedia gives a similar statement:

https://en.wikipedia.org/wiki/Libration

  “ Diurnal libration is a small daily oscillation due to Earth's rotation, which carries an observer first to one side and then to the other side of the straight line joining Earth's and the Moon's centers, allowing the observer to look first around one side of the Moon and then around the other—since the observer is on Earth's surface, not at its center. It reaches less than 1° in amplitude.^[3] ”

Pointing Towards the Center of the Earth

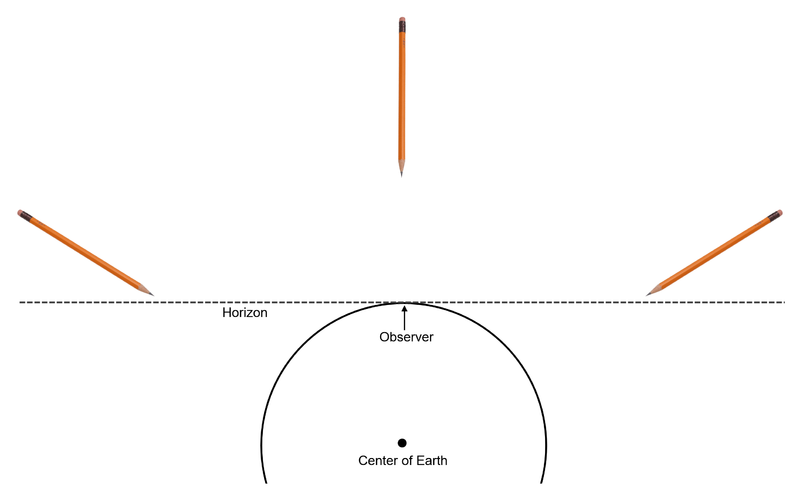
After accepting that the Moon would turn minimally to perspective, our opponents next argue that a 1° rotation of the Moon is enough to cause the effect, since in an angular diameter calculator "one degree at a distance of 238,900 miles subtends to 4170 miles of horizontal space." As reply to this, it is questioned how, exactly, such a small degree of rotation could cause the great magnitudes of the Moon Tilt Illusion seen in the diagrams at the start of the page. It is further pointed out that the Moon would not tilt upwards to perspective at all in a scenario where the Moon is tidally locked with the center of the Earth and a change of perspective occurs as result of the observer's displacement from the center.

In the below diagram a pencil is pointing at the center of the Earth. Due to displacement from the center, the observer will see the top side of the pencil when it is near the horizon, observing that it points downwards when at the horizons on either side of the observer. Although the diagram is not to scale, and the pencil rotation depicted is much greater than 1° due to the close proximity to the Earth, we can see that the Moon would not point upwards in relation to the nearest horizon, as would be required of a perspective explanation.

Hallway Perspective

Another perspective example is given in the way of standing in the middle of a hallway. In The Moon Tilt Illusion (Archive) we read a description:

The above scenario involves standing in the middle of a long hallway, and observing that the ceiling above you is horizontally parallel and that the the angles of the ceiling at either end are tilted to perspective. The movement of the Moon is claimed to be just like traveling down or up the length of the ceiling of a hallway, and will change to perspective like the angled corners of the ceiling at either ends. At one end of the hallway the Moon is angled upwards in one direction, when it is over you it will be horizontal, and then when it is down on the other end it will be angled downwards.

However, the example involves a celestial body that is close to the earth, radically changing distance to the observer in relation to their distance above the Earth. Under the Round Earth model the Sun and Moon are essentially the same distance from the observer at all times, and so will not change much to perspective. In contrast to the Round Earth model, the edges of the hallway ceiling in the example are not the same distance from the observer at all points. The points along the edge of the hallway ceiling change radically in distance to the observer.

The above example would predict that we would see different sides of the Moon as it approaches and recedes from the observer. And if one were to alternatively suggest that the physical features of the Moon ball's face are always turning to point at us as it moves down the hallway, it would then predict a daily movement of the shadow across the physical features of the Moon's face, none of which we see in reality. We see again that the argument made is specious and unrelated to what is claimed by the Round Earth Theory's astronomical model.

Ball Experiment

Another attempt at showing that the matter may be caused by perspective takes the form of a ball experiment performed with the Moon in the background. Since it is possible to use a close range perspective effect with a ball in the near field to get it to point in a variety of different directions, it is concluded that this what must be happening with the Moon.

In a MetaBunk thread Mick West views a Moon Tilt Illusion and performs the following: (Archive)

The red arrows in the image above depicts the directions of light for the bodies in question, as seen from a far vantage point. The Moon (top red arrow) is unexpectedly pointing above from the Sun while the ball on a post (bottom red arrow) is pointing towards the Sun, as would be expected. It is expected that bodies illuminated by the Sun would point towards it.

Mr. West then approaches the ball and angles the camera close and right up to a ball on a post to get it to point away from the Sun like the Moon does:

Mr. West concluded that this is what must be happening with the Moon.

The author uses a close-range perspective effect to match the Moon. There is little doubt that holding something very near to one's face or camera can create lots of angles. Yet, in the first far distance scene the illuminated portion of the ball points at the Sun. It is not until the observer gets up close to the ball, to a special carefully selected position beneath it, that the ball is able to point away from the sun, in a similar direction as the Moon.

The fallacy in this example is that under RET the Moon is not a small ball very close to the observer — a false analogy. In RET the Moon is far from the observer and maintains its distance from the observer and Sun at all times — it is not subject to close range perspective effects. The Moon does not radically change its distance from the observer at any time. Perspective affects orientation less and less with greater distances.

The photographer's action of moving close up to the ball, looking at its underside and invoking close range perspective effects so that the ball is tilted upwards, is inapplicable to RET. We view the same side of the Moon at all times. In order to tilt upwards to perspective a body would need to change orientation and angle, just as if we were lifting any body upwards in front of us.

We again see repetitions of the fallacies above, and the failure to provide a proper geometric explanation for the Moon Tilt Illusion.

Garage Experiment

Mr. West provides a similar perspective example in a garage experiment video.

In this example, like the previous example, we are looking at the ball from the underside, and so the moon-ball is tilted upwards.

One comment in the video states: "This is a pretty neat illusion, and definitely one that makes flat-Earthers think they've somehow "cracked the code" when in reality they're just misunderstanding geometry."

In truth, the opposite is the case. If the Moon were shifting to perspective to such a degree in a daily basis, by tilting up and down it would necessarily manifest such effects as a changing of angles and the shifting view of different views of the sides of the surface, or shifting of the shadow in relation to surface features. Yet, this is not known at all. The perspective argument is an explanation which has been made up for the purpose, known nowhere else in observational astronomy. Unless Mr. West is proposing that the Sun and Moon are small bodies moving very close over his head like in the models he constructs, and where we see different sides of the Moon, changing angle as it moves as result of perspective effects, the comparison must be seen as nothing other than a fallacious misunderstanding of geometry.

Celestial Sphere

Another explanation for the Moon Tilt Illusion involves a "Celestial Sphere" in the sky, upon which straight lines become curved.

In the paper The Moon Tilt Illusion (Archive) by Adrea and Alan Myers, the following is stated:

  “ The moon tilt illusion is not described in astronomy textbooks because astronomers know that straight lines in object space become great circles on the celestial sphere. Minnaert [5] gives only a passing reference: “...the line connecting the horns of the moon, between its first quarter and full moon, for instance, does not appear to be at all perpendicular to the direction from sun to moon; we apparently think of this direction as being a curved line. Fix this direction by stretching a piece of string taut in front of your eye; however unlikely it may have seemed to you at first you will now perceive that the condition of perpendicularity is satisfied”. An article by Sch¨olkopf [8] documents the illusion in an experiment involving 14 subjects by having them indicate their expectation of how the moon’s illumination should be oriented with respect to the position of the (visible) sun. He reports that an average discrepancy of 12◦ is perceived by the subjects between the observable versus expected orientation of the moon’s bright limb. Schott’s website entitled “ ‘Falsche’ Mondneigung” (‘False’ Moontilt) [9] is devoted to the moon tilt illusion, and features illustrations and useful links. Schott correctly proposes to quantify the effect by comparing the observed tilt angle with the angle from horizontal of the line connecting the moon and sun, but an error in geometry leads to an incorrect expression for the expected tilt. A paper by Glaeser and Schott [2], approaching the phenomenon via the principles of photography, show that the magnitude of the illusion could in theory be measured through comparison of a close-up shot of the moon with a photograph containing both sun and moon, with the camera directed in a specified direction between them (although no equations are given). However, as they point out, in practice it is not feasible since even a wide-angle lens cannot capture both sun and moon in a photo with azimuth differences for which the illusion can be most clearly observed (between 90◦ and 180◦). Berry[1] proposed using a star chart, which is a zenith-center stereoscopic projection of the celestial sphere onto a flat surface, to define the moon tilt illusion as the angle between the projected great circle and a straight moon-sun line drawn on the same chart “mimicking how we might see the sky when lying on our back looking up”. Clearly, there exists a lack of consensus in the literature about the explanation of the moon tilt illusion and disagreement about the best way to describe it.

~

Astronomers rely upon the celestial sphere model for maps of the sky because locations of stars and constellations depend only on their right ascension and declination. For the topocentric model used for the sun and the moon, location is specified by azimuth and altitude. All objects in the sky are assumed to be located at the same distance from the observer, as if pasted upon the surface of an imaginery sphere surrounding the observer. Astronomers, for whom the celestial sphere model is a basic tool for mapping the stars, are not surprised by the apparently curved path of light from the sun to the moon because they know that straight lines in 3-D object space are transformed to great-circle arcs on the imaginary celestial sphere. ”

We are told that straight lines become curved when looking into the sky because of the "celestial sphere" which exists above our heads.

3D Model Example

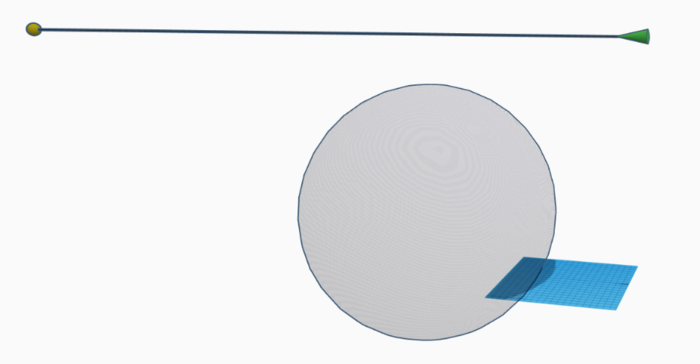
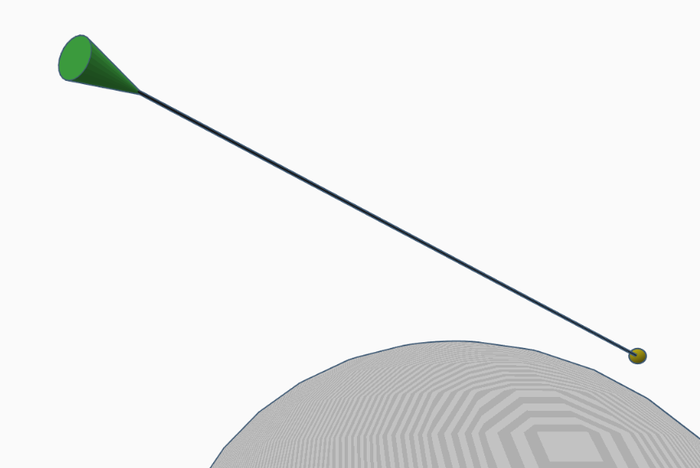
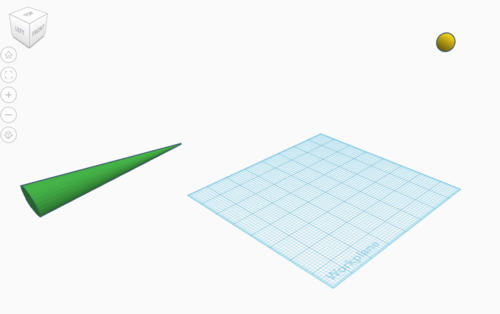
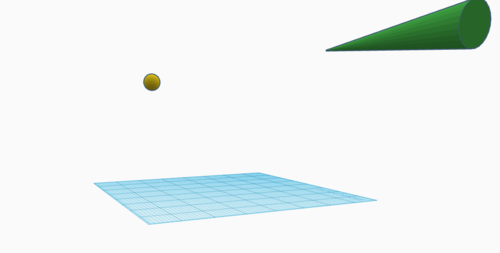
A demonstration against this concept may be found in any 3D modeling program. Create a long straight line and place the camera at various positions and distances from it. At what point does the straight line become curved? The answer we have found is: At no point. A straight line in the distance will always be straight.

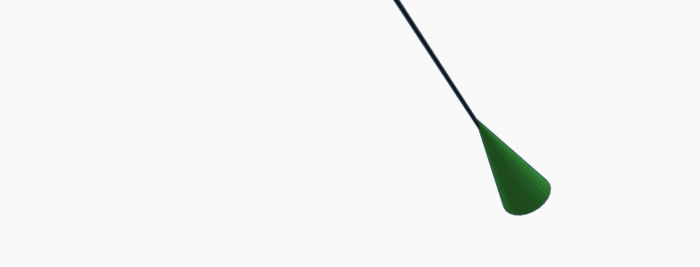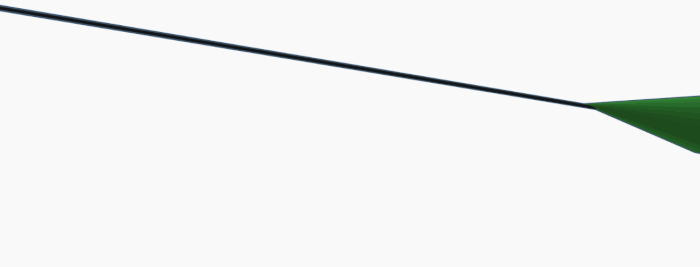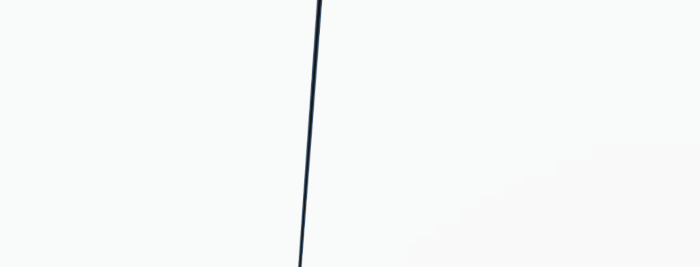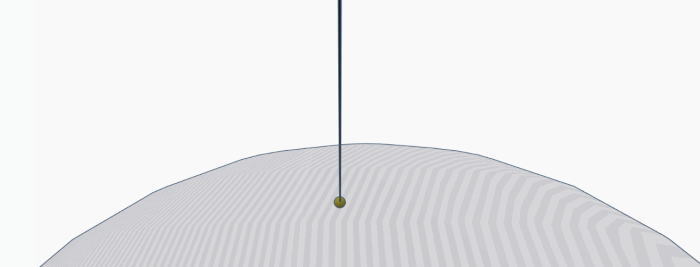
Alternatively, if the line were a green cone pointing at a yellow ball, the green cone would always point at the yellow ball.

In the above we took a picture of a 3D scene in various positions. With various positions and greater and greater distances from the scene, the cone will always point at the ball. One must wonder, why does the arrow always point at the ball? The assertion that arrows would not point at the things they are pointing at also implies that if you took a picture of any line in 3D space, that it would appear curved on the picture.

On this topic we receive arguments such as "What you are failing to consider is that the night sky is not a euclidean space; it's a curved surface." But just what is being described? In RET the space above and around it is not a "curved surface". It's not a surface at all. What reason is there to think that we would see it as a surface around us where straight lines become curves? This can only be seen as wild imagination from a desperate position.

Hence, the concept of a celestial sphere is seen to be a contrived concept bearing no relation to the 3D space which the Sun-Earth-Moon systems of astronomy are said to exist in. As there is no sphere around the Earth in RET upon which straight lines may become curved, it is again found that a geometric explanation for the Moon Tilt Illusion is unavailable. Astronomers disagree among themselves on the cause, with some speculating on a planetarium-like celestial sphere which does not exist in the physical Sun-Earth-Moon system.

Celestial Sphere Project

Our experts on astronomy provide us with a Moon Tilt Illusion scene cut into a 'Celestial Sphere' to give us a better idea on "how things work in 3D space." Straight lines become curved on the Celestial Sphere.

(Source Images)

Unfortunately, this example comes with a fictitious premise. The sky is not a sphere which the Sun, Moon, and lines of light rays rest against to cause artificial curvature. Straight lines will be straight in space above us, not 'curved on a celestial sphere'.

If the Sun and Moon can be connected in such a manner, it merely provides a small clue for the underlying mechanism, and does not imply that there is a "celestial sphere" above the Earth where "straight lines become curved". Such a mechanism is entirely unexplained and nonphysical in the Round Earth Theory.

String Experiment

Along the same lines as the above, we are given reference to "string experiments" in which the direction of the Moon's illuminated portion is able to be connected to the sun with a string.

Credit: Bobby Shafto

It has been argued that the string experiment shows that the bodies do actually point at each other. An illusion of some type is occurring and the string experiment "breaks the illusion," demonstrating that the illuminated portion of the Moon is actually pointing at the Sun. If it was not pointing at the Sun then it would not be possible to hold a straight piece of string along that path.

As a reply to this, consider the following scenario:

You are laying down on the ground on your back, facing upwards, and at the edges of your vision see the top of a pine tree on one side of your vision, and the top of a cabin on the other. You take out a string and connect them together across your vision. Have you proved that the tree is pointing at the cabin?

If you are laying down on the ground on your back and see the Moon pointing upwards on one side of your vision and see the Sun setting at the horizon on the other, a string connecting the two will no more prove that the Moon is pointing at the Sun than it would prove that a tree is pointing at a cabin. When you lay on your back you can see 190 degrees of space¹. Just because an object at one side might be pointing "up" at another object at the other side, it doesn't mean that they are pointing at each other.

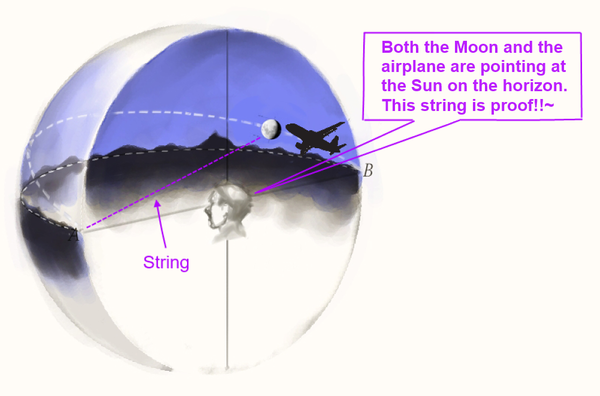
When wrapped around the observer, this panoramic view of the moon tilt illusion:

Turns into this:

Art Credit: Todd Lockwood

In the above example both the Moon and airplane are on opposite sides of the Sun near point B. The Sun is on the horizon at point A. The Moon and airplane are not actually pointing at the Sun. The string merely connects them two dimensionally across a 'sphere of vision' exactly like the tree-cabin example.

If the airplane was actually pointing at the Sun in the above example, then when looking at the airplane face on, with the Sun on the horizon to your back, you should see the airplane pointed at you and tilted downwards towards the opposite horizon behind you. The same would also apply for the Moon. If the Moon were pointing at the Sun then when you face the Moon its illumined portion should point downwards at the Sun at the horizon behind you, just as an airplane would. Thus, we see that this assertion that the string experiment demonstrates that an illusion is occurring and that bodies are pointing at each other is erroneous. The string experiment may suggest that object positions and straight line paths behave as if they are curving on a dome of some manner, which may provide us with a clue in deciphering the nature of our world, but it does not demonstrate absolute directions of bodies.

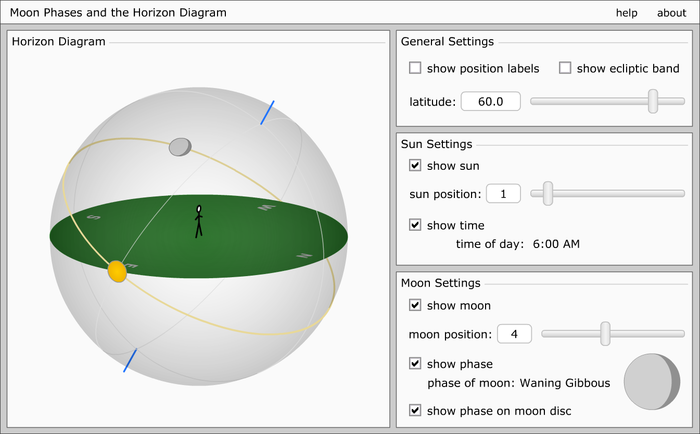
A fish-bowl type simulation of the Moon Tilt Illusion can be seen in University of Nebraska-Lincoln's Moon Phases and the Horizon Diagram (.swf Archive) - "Provides a method of learning the correlation between the phase of the moon, the time of day, and the position of the moon in the sky."

Footnotes

¹ "our eyes sit in the front of our head, allowing us to see about 60 percent of world in front of us with both eyes, at the compromise that we can only see at maximum about 190 degrees around us (Block 1969; Wolfe 2006)" – Human Spatial Navigation, 2018, p.73

Curving Lines on Celestial Sphere

Referring back to a Celestial Sphere explanation, it is next more explicitly claimed that lines curve on a Celestial Sphere around us, and it is again insisted that we merely need to "do the string experiment" to see that the paths connect, showing the existence of this sphere.

The claim that lines curve on the Celestial Sphere above us is to claim that if we shine a laser into space that we will see it wrap around the dome and hit the opposite horizon.

If Dexter fires his laser beam into the sky and into space, will we see it 'wrap around' on the Celestial Sphere and hit the opposite horizon?

We are obliged to answer in the negative. The laser beam will not wrap around to the opposite horizon. We again see that the mechanism of a 'Celestial Sphere' is nonphysical and unexplained.

At amazingsky.com we find the following (Archive):

Caption: "The MSM’s bright laser pointer is useful for aiming the tracker at the North Celestial Pole, located about a degree away from Polaris in the direction of Alkaid, the end star in the Handle of the Big Dipper or Plough."

If the laser could continue forever into the distance, would it curve around on the Celestial Sphere and hit the horizon?

Predictive Equation

In The Moon Tilt Illusion (Archive) Professor Myers provides equations which can be used to predict the Moon's tilt in the Moon Tilt Illusion. Some have attempted to reference these equations as a piece of evidence that the Moon Tilt can be explained under the Round Earth Theory. Assessing the scheme of the model used, however, shows that it is not a Round Earth model.

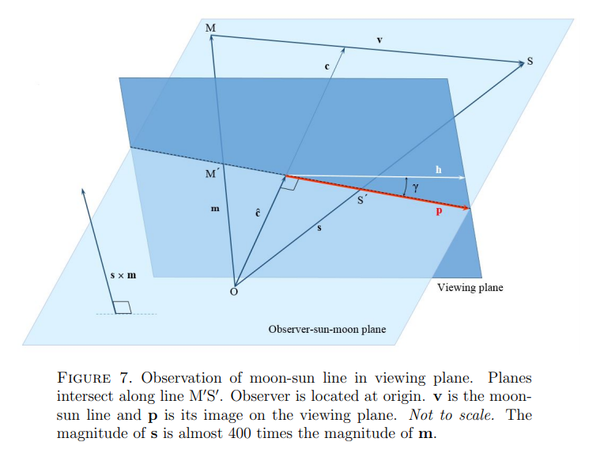
Viewing Plane

In Professor Myers' model the distant Moon and Sun are projected onto a "viewing plane" near the observer. Figure 7 on p.8 shows a diagram of this model:

In the above caption it is stated of the Moon and Sun that the "image is on the viewing plane". Is there a viewing plane above our heads in RET where bodies are projected upon? As we are not taught that there is a "viewing plane" close above our heads where the celestial bodies are projected, we must question the premise of the calculations.

Why is a "viewing plane" necessary? We see that the model is unrelated to the systems which are said to be in place.

Celestial Sphere

Previously, we had read that Professor Myers told us about the curving of light on the celestial sphere as cause of the Moon Tilt Illusion. He states:

  “ Astronomers, for whom the celestial sphere model is a basic tool for mapping the stars, are not surprised by the apparently curved path of light from the sun to the moon because they know that straight lines in 3-D object space are transformed to great-circle arcs on the imaginary celestial sphere. ^[2] ”

  “ The scientific explanation is based on the projection of a straight line onto the surface of a sphere ^[3] ”

  “ The moon tilt illusion is not described in astronomy textbooks because astronomers know that straight lines in object space become great circles on the celestial sphere. ^[4] ”

At the bottom of p.2 of his paper The Moon Tilt Illusion (Archive) Professor Myers explains that the celestial sphere is used as an axiom in the equations for the predictive portion:

  “ Our aim is to derive an equation for the magnitude of the moon tilt illusion that is straightforward to apply to all configurations of sun and moon in the sky. The viewer’s expectation for the direction of incoming light is modeled using vector geometry, which is appropriate for treating 3-D straight lines such as the sun-moon light ray. Analyzing an illusion may seem trivial but the explanation of the moon tilt illusion requires knowledge of the perspective projection basis of human vision, vector algebra, and geometrical concepts such as orthographic projections, the celestial sphere, and geodesics. ”

Once we consider all of the above, including geometrical concepts such as the celestial sphere, upon which the celestial bodies are assumed to be resting on at different angles and where straight lines become curved, we can explain the Moon Tilt Illusion.

Distance to Sun and Moon Not Used

On p.9 we see statements that the distances to the Sun and Moon are not used and are avoided.

  “ The value of the angle α is the same for the vectors m, s and z or their corresponding unit vectors, which are used in Eq. (11) to avoid having to know the actual distances of the moon and the sun from the observer. The moon and sun unit vectors... ”

Doing the Math

At the 42 minute mark of a video titled Moon Tilt Illusion (Doing the Math), which looks at the math in the Myers paper, the narrator explains that the distance from the earth to the sun does not matter, and that in this model the illuminated portion of the Moon will point in the same direction regardless of the distance of the Sun to the Earth. The illuminated portion of the Moon will always point in the same direction, whether the Sun is 0 or 100,000,000 units of measurement away from the observer.

A Simple Challenge

To those who do think that this model does represent a Round Earth model; a simple exercise is to go through the document and point out the page or section where the distance from the Earth to the Sun or the Earth to the Moon is expressed in the equations. We have found that the distances do not appear anywhere in the equations.

From all of the content above we have seen that distances do matter. There is a perspective difference between moving bodies which are close by and moving bodies which are millions of miles away. A Round Earth model should have Round Earth properties. Since the above predictive model does not use the Round Earth distances, and instead appeals to 'viewing planes', 'celestial spheres', and undefined distances, it cannot be a Round Earth model. The required geometry could be seen to act more as evidence against than for.

Conclusion

The Flat Earth Theory provides a geometric explanation for the Moon Tilt Illusion and the dome-like movement and orientation of the celestial bodies in the sky. Its astronomical model directly predicts the tilting of the Moon's phase above and away from the Sun.

The Round Earth Theory, in contrast, has difficulty with providing a working explanation for the Moon Tilt Illusion. Straight lines in normal space should cause the illuminated portion of the Moon to point at the Sun. The explanations for this appeal to questionalbe perspective explanations and lines which curve on a "celestial sphere". A perspective explanation would require the Moon to shift in angle and orientation upwards in order to tilt upwards, to which we should able to see different sides of the Moon, or we should otherwise see movement of the shadow on its tidally locked face; whereas a celestial sphere explanation is entirely immaterial and without a mechanism. In complete contradiction to popular dogma, it is the Round Earth Theory which has geometric difficulties, lacking explanatory power for common observations.

Topics

• Electromagnetic Acceleration - EA Main Page

• Lunar Eclipse due to Electromagnetic Acceleration - The Lunar Eclipse occurs when the Moon moves beyond the Sun's light
• Moon Tilt Illusion - Illuminated portion of the Moon does not point in expected direction
• Celestial Sphere - Lines become warped on a 'Celestial Sphere'
• High Altitude Horizon Dip - Horizon dip at high altitudes may suggests a bending of light

Sunrise and Sunset - Sunrise and Sunset Main Page