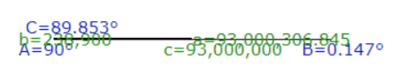Difference between revisions of "Moon Tilt Illusion Supplement"
Tom Bishop (talk | contribs) |
Tom Bishop (talk | contribs) |
||
| Line 1: | Line 1: | ||
| − | The '''Moon Tilt Illusion Supplement''' page addresses the common counterarguments and fallacies related to the [[Moon Tilt Illusion]]. Specifically, the String Experiment alleges that a string will demonstrate the direction of the Moon, the Ball Experiment alleges that a small ball with matching phases shows that | + | The '''Moon Tilt Illusion Supplement''' page addresses the common counterarguments and fallacies related to the [[Moon Tilt Illusion]]. Specifically, the String Experiment alleges that a string will demonstrate the direction of the Moon, the Ball Experiment alleges that a small ball with matching phases shows that a perspective illusion is occurring, and Higher Sun Argument says that the Moon sees the Sun from a higher position than the observer. |
==String Experiment== | ==String Experiment== | ||
Revision as of 05:32, 7 March 2021
The Moon Tilt Illusion Supplement page addresses the common counterarguments and fallacies related to the Moon Tilt Illusion. Specifically, the String Experiment alleges that a string will demonstrate the direction of the Moon, the Ball Experiment alleges that a small ball with matching phases shows that a perspective illusion is occurring, and Higher Sun Argument says that the Moon sees the Sun from a higher position than the observer.
String Experiment
As justification for the Moon Tilt Illusion we are told to take a piece of sting and to hold it across the ecliptic, the path across the sky the Sun and Moon cross daily, and observe that the direction of the Moon appears to connect to the Sun in the sky.
It has been argued that the string experiment shows that the bodies do actually point at each other. An illusion of some type is occurring and the string experiment "breaks the illusion," demonstrating that the illuminated portion of the Moon is actually pointing at the Sun. If it was not pointing at the Sun then it would not be possible to hold a straight piece of string along that path.
As a reply to this, consider the following scenario:
- You are laying down on the ground on your back, facing upwards, and at the edges of your vision see the top of a pine tree on one side of your vision, and the top of a cabin on the other. You take out a string and connect them together across your vision. Have you proved that the tree is pointing at the cabin?
If you are laying down on the ground on your back and see the Moon pointing upwards on one side of your vision and see the Sun setting at the horizon on the other, a string connecting the two will no more prove that the Moon is pointing at the Sun than it would prove that a tree is pointing at a cabin. When you lay on your back you can see 190 degrees of space1. Just because an object at one side might be pointing "up" at another object at the other side, it doesn't mean that they are pointing at each other.
Footnote
- 1 "our eyes sit in the front of our head, allowing us to see about 60 percent of world in front of us with both eyes, at the compromise that we can only see at maximum about 190 degrees around us (Block 1969; Wolfe 2006)" – Human Spatial Navigation, 2018, p.73
Fish Bowl Diagram
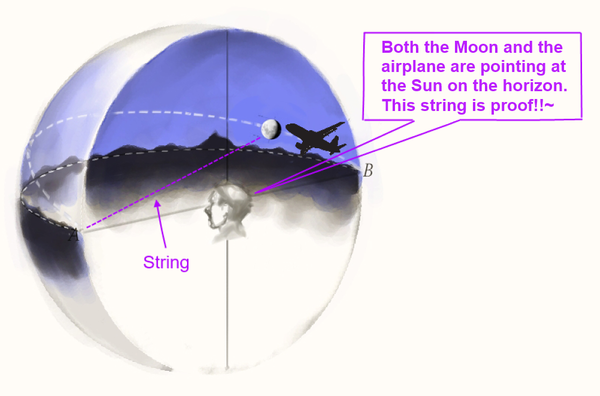
When wrapped around the observer, this panoramic view of the moon tilt illusion:
Turns into this:
In the above example both the Moon and airplane are on the ecliptic and on opposite sides of the Sun near point B. The Sun is on the horizon at point A. The Moon and airplane are not actually pointing at the Sun. The string merely connects them two dimensionally across a 'sphere of vision' exactly like the tree-cabin example.
If the airplane was actually pointing at the Sun in the above example, then when looking at the airplane face on, with the Sun on the horizon to your back, you should see the airplane pointed at you and tilted downwards towards the opposite horizon behind you. The same would also apply for the Moon. If the Moon were pointing at the Sun then when you face the Moon its illumined portion should point downwards at the Sun at the horizon behind you, just as an airplane would. Thus, we see that this assertion that the string experiment demonstrates that an illusion is occurring and that bodies are pointing at each other is erroneous.
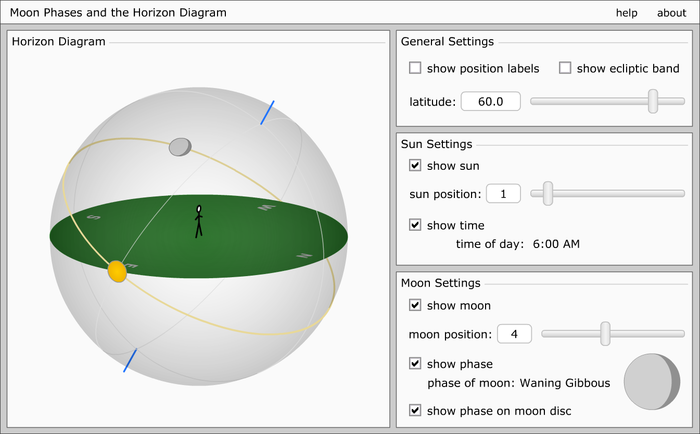
University of Nebraska Simulation
A fish-bowl type simulation of the Moon Tilt Illusion can be seen in University of Nebraska-Lincoln's Moon Phases and the Horizon Diagram (.swf Archive) - "Provides a method of learning the correlation between the phase of the moon, the time of day, and the position of the moon in the sky."
In this simulation the direction of the Moon follows the ecliptic as it progresses across the sky. Adjusting the sliders it is seen that when the Moon is rising its phase points upwards, at Midmoon the phase makes a right angle in the sky, and at setting the phase points downwards, along that yellow ecliptic.
In the above image, if the observer in the scene were to hold a water bottle at arm's length up against the Moon, it would appear that the water bottle was pointing upwards into space away from the Sun. And per the 'string experiment', if the observer were to take a string and connect the Moon to the Sun across the ecliptic, the Moon's phase would connect with the Sun.
String Experiment Not Reliable
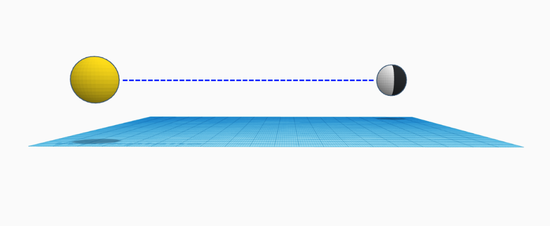
In the below overhead scene we have a Moon misaligned from the Sun.
Changing the view to another position, we can connect a string between the two. Does this ability to connect a string prove that the Moon is pointing at the Sun?
We thus see that the string experiment is simply unable to distinguish whether a body is pointing at something else. It may provide a clue showing the adjacent nature of the phase with the ecliptic, but it does not show the absolute direction of bodies.
Higher Sun Argument
As response to the Moon Tilt Illusion it is sometimes argued that the illuminated portion of the Moon is misaligned to the Sun since Sun's light is parallel and hitting the Earth-Moon system as a whole from the side, and so the Moon will see a higher Sun than an observer on the Earth. The Sun will appear from a different position in space to the Moon, causing the Moon's illuminated area to point to a position above the Sun that we see from Earth.
In contrast to this argument, the angles are not perfectly parallel under the Round Earth system. When assessing the parallax displacement between an observer on Earth and an observer on the Moon the Sun will be in essentially the same point in space.
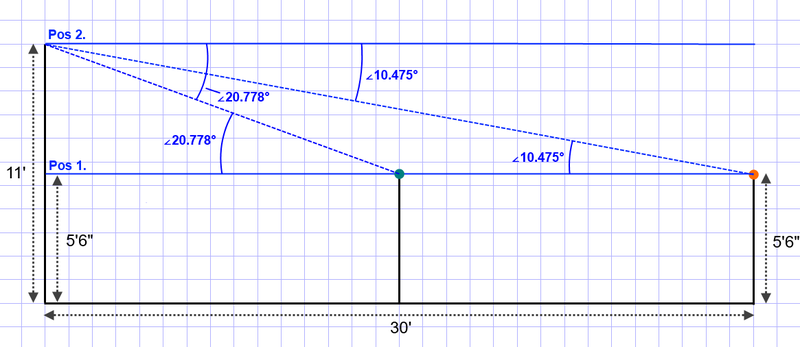
Angular Diameter Example
As an example, if your eye is at an altitude of 5 feet, 6 inches, and there is an object, say a Green Ball on a post 15 feet away from you at the same altitude, and an Orange Ball on a post 30 feet away from you, which are both also at altitudes of 5 feet, 6 inches, then the position of both the green and orange balls will be parallel on the horizontal. The path to those objects will be at your eye level.
Instead, lets say that you step on a boulder that is 5 foot, 6 inches in height. The new altitude of your eye is 11 feet in height. We know that the green and orange balls are on posts which are 5 ft 6 in below that 11 foot elevation where it would need to be to be parallel to the eye.
In order to find the position of the green and orange balls in degrees below eye level, we can use an angular diameter calculator to calculate the missing space above the green and orange ball, to determine how far below eye level those balls will be to the observer.
For the Green Ball, 5.5 feet of vertical space at 15 feet produces an angular diameter of 20.778 degrees. For the Orange Ball, 5.5 feet of vertical space at 30 feet produces an angular diameter of 10.475 degrees.
Diagram
We see that as a body moves further from you, it gets closer to your eye level, and displaces fewer degrees in space.
Adopting the above to the Earth and Moon in the RE system, we had from the previous diagram an observer seeing the Sun parallel on the horizontal at eye level, midway setting into the horizon. The Moon was over the observer. In order to calculate the displacement of the Sun in the between observers on Earth and the Moon we may use the angular diameter calculator with the distances involved in miles.
Input
g: 238900
r: 93000000
Output
a: 0.147 degrees
If the observer was able to move from the Earth to the Moon the Sun would be seen from essentially the same position in space. The diagram of the Sun appearing in two different places may be misleading, as it does not show the entire scene and the angles are not exactly parallel to the Sun.
Triangle Calculator
Next, we look at a triangle calculator, which will compute the missing values in a triangle:
We put in the distances in miles to the RE Moon at Side b, the distance to the RE Sun at Side c, and 90 degrees at the observer at Angle A. This produces the following:
We see a very thin Right Scalene Triangle diagram. We see that the angle displacement of the Sun at Angle B is 0.147 degrees, as previously seen in the angular diameter calculator. We also see that Moon at Angle C is pointing downwards at 89.853 degrees; not exactly parallel to the baseline.
The Sun is not actually shining on the Earth and Moon in a parallel manner, but at slightly different angles.
Triangle Diagram
Now, consider the above thin Right Scalene Triangle diagram which was generated by the Triangle Calculator:
If the object at Angle C Moon position was a Green Arrow pointing at the center of the Sun (Angle B), and if we could see both the Green Arrow and Sun at once in our field of vision (essentially the whole scene), would the the Green Arrow point at the Sun?
This should be true regardless of the shape of the triangle, and demonstrates the expectation that the illuminated portion of the Moon should point at the Sun when viewed simultaneously. The Green Arrow should not point at a different spot in space.
Alignment with Sun Center
Finally, it is suggested that since the Sun is so large and the rays are essentially parallel to the relatively small Earth-Moon system at their distant location from the Sun, that the illuminated portion of the Moon is not necessarily pointing at the center of the Sun (restatement of premise).
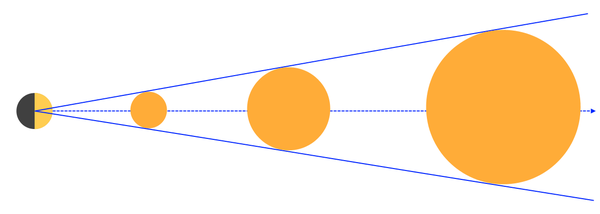
Consider a situation where we have only have two bodies: The Moon and a Sun. The Sun starts as the size of the Moon (or smaller). The illuminated portion of the Moon will point at the center of that Sun. If the Sun continuously recedes in distance from the Moon and grows in size geometrically in a linear fashion, will the Moon ever not point at the center of the Sun?
Hence we see that, with only two bodies, the Moon will always point at the Sun's center, regardless of distance or size. There is no real reason for it to point anywhere else. From the position of the central hotspot upon the Moon's surface it will see the Sun directly 'overhead', and will be illuminated from directly 'overhead', regardless of how the Sun recedes or grows. The addition of an Earth somewhere around the Moon should not matter in regards to where the illuminated portion of the Moon points.